
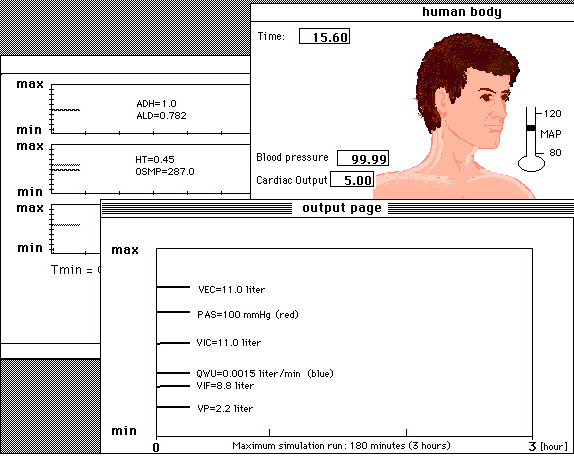
Figure 10.1. A screendump of the computer simulation program FLUIDS designed with MacTHESIS.
This chapter discusses:

Students have 'a testee' at their disposal so to speak, with which
they can do a series of experiments. Thus it is possible, for example,
to imitate excessive thirsting, sweating, or excessive loss of water and
salt. A change in the oxygen and carbon dioxide fraction during breathing
can also be simulated. Furthermore disorders in the acid-base balance can
be simulated and the function of the kidney .
Beside interventions with the potential of making the patient ill,
therapeutic interventions are also possible, such as applying infusions
of various compositions in order to give extra oxygen for breathing or
the administration of a diuretic. It is also possible to do the so-called
glucose tolerance test (GTT). Despite the complexity of the model it has
been observed that students can easily find their way in this computer
simulation program. In this simulation the emphasis is on free investigation
in order to develop in the students an overall picture of the way the human
body works as a complex physiological dynamic system.
The model by Ikeda and co-workers consists of the following sub-systems:
circulation, respiration, kidney function, and the intra- and extra-cellular
fluid compartments. In all the model consists of 30 integral equations
plus other algebraic equations, totalling more than 270 variables. Water
and salt regulation varies greatly among individuals and also between the
sexes. In this model (a young man of 55 kg) the body fluids are divided
into three major groups: the intracellular (20.0 liter), the interstitial
(8.8 liter) and the intravascular compartment (2.2 liter). The relatively
small amount of transcellular water (brain fluid, fluid in the stomach
and intestinal canal, eye compartment fluid and such) is not taken into
account and only plays a small role in the total weight. The extracellular
volume is 11.0 liter and the blood volume 4.0 liter. The composition of
blood plasma, interstitial fluid and extracellular fluid, as well as that
of the dissolved ionized matter and the molecular dissolved matter of gasses,
is very specific.
The normal values of ion concentrations and others in the extracellular
and intracellular fluid compartments in the computer simulation program
FLUIDS are:
| Extracellular concentrations: | Intracellular concentrations: | |
| Sodium Na+ | 140 mEq/l | 10 mEq/l |
| Chlorine Cl- | 104 mEq/l | 4 mEq/l |
| Potassium K+ | 4.5 mEq/l | 140 mEq/l |
| Calcium Ca++ | 5 mEq/l | << 0.001 mEq/l |
| Magnesium Mg++ | 3 mEq/l | 58 mEq/l |
| Bicarbonate HCO3- | 24 mEq/l | 10 mEq/l |
| Phosphate PO4--- | 1.1 mEq/l | 75 mEq/l |
| Sulphate SO4-- | 1 mEq/l | 2 mEq/l |
| Glucose | 6 mosmol/l | 0 mosmol/l |
| Urea | 2.5 mosmol/l | 2.5 mosmol/l |
There are many regulation mechanisms to keep changes in the concentrations in the extra- and intra-cellular fluid compartments as small as possible. The kidney plays an important part here, by regulating the volume and the colloid osmotic pressure of the fluid compartments. In addition there are regulation mechanisms for the degree of acid, for the stabilization of the blood gas pressure of O2 and CO2, for the stabilization of the ionic composition, and for the stabilization of the glucose percentage. The model includes seven parts that can be distinguished, and the relations between them are shown in Figure 10.2. This figure also indicates some interventions which are possible in this model. The different parts of the model as shown in Figure 10.2 are:
Cardiovascular system
The cardiovascular system has been minimized to a functional unit for
the cardiac output and the mean arterial pressure, which in this model
depend only on the blood volume, elasticity of the vascular system, and
the peripheral resistance. Because the cardiovascular system has been so
minimized, simulation of a cardiovascular affliction is not easy with this
model.
Respiratory system
The regulating system of respiration is a functional unit in which
ventilation depends on the pH, the CO2-, and the O2-pressure in the arterial
blood. The pH of the blood is determined by the percentage of freely dissolved
CO2 and the percentage of HCO3-. The equation of Henderson-Hassalbalch is valid
here. The hemoglobin buffer system can keep the pH of the blood constant
by the intake or release of H+-ions, although there are situations when
a large or fast increase or decrease of H+-ions takes place.
 book)
book)
 book)
book)The pH in the blood is largely determined by animo acids, composed of proteins. This buffer system of H+-ions regulates the pH around 7.4 (iso-hydric point). Another buffer system for the pH is the bicarbonate buffer. The proper functioning of this system requires the CO2 surplus to be adequately removed via the lungs.
Extracellular fluid compartment
This part of the model simulates the regulation of the fluid volumes.
The dissolved ion concentration in the extra-cellular fluid compartment
is closely connected to the quantity of water taken in and excreted by
the body. The fluid volume in the extra-cellular compartment is determined
by the quantity of water which is administered orally or intravenously
and by the loss of fluid in the form of urine and sweat. The intake of
concentrations of the dissolved matter held in this system usually comes
via food from the stomach and intestines. Intravenous administration through
an infuse is also possible in this model. All intakes have been normalized
on an average of 24 hours with certain referential values per minute.
Intracellular fluid compartment and electrolytes
In this part of the model Ikeda and co-workers have classified the
intracellular fluid volume, the osmotic active substances in the intra-
and extra-cellular compartments and the intra-cellular acid-base balance,
among others sodium, potassium, chlorine, glucose, ureum and mannitol.
The change in the extra cellular sodium quantity (ZNE) is represented in
the model as follows:
d(ZNE)/dt = intake by Na(YNIN) - excretion of Na(YNU) + increase of Na, in exchange for H+-ions in the cell
and the change of the quantity of potassium in the extra-cellular compartment (ZKE) like this:
d(ZKE)/dt = intake of K(YKIN) - excretion of K(YKU) + increase of K, in exchange for H+-ions in the cell - K which goes into the cell in connection with glucose metabolism and insulin secretion.
The ureum reabsorption mechanism in this model is passive while it is assumed that about 60% of the filtered quantity will eventually be excreted. The plasma osmolality (OSMP) depends in this model on the sodium, potassium, chlorine, glucose, ureum and mannitol concentrations, and on a constant factor for the other osmotic active substances.
The kidney
The renal excretion for bicarbonate, calcium, magnesium, phosphate,
and organic acids are in this model functions of the glomerular filtration
velocity and the concentrations of these ions. A quantity of fluid from
the plasma is filtered through the glomerulus. The blood pressure is the
driving force in this model. The largest part of the filtrate returns then,
selectively, into the plasma in the peritubular capillaries.
The transport of potassium and sodium is active, going against the concentration gradient as well as the electric gradient. The negative ions, like chlorine, diffuse passively, with the exception of bicarbonate. The transport of water only happens if there is an osmotic driving power. The model attempts to keep the osmolality of the plasma constant. Chemoreceptors play an important part here. The active transport of ions and the transport of water is influenced by hormones. In this model the active transport of sodium is aided by aldosterone which retains (reabsorbs) sodium and excretes potassium. The excretion of water can be checked by the antidiuretic hormone.
 book)
book)Construction of the model
Below the formulation of the most essential parts of the various blocks
is described.
The circulation
The cardiac output (QCO) is only dependent on the blood volume (BV)
and determines together with the total peripheral resistance (RTOT) the
mean arterial pressure (PAS).
The fluid volumes
The plasma volume (VP) is determined by the quantity of water which
is administered intravenously (QVIN), the quantity which is taken in by
the body orally (QIN), the quantity of water which leaves the body in the
form of urine (QWU) and also by a.o. the quantity of water which leaves
the body through sweating (QIWL) and that which is formed metabolically
(QMWP).
The blood volume (VB) and the extracellular fluid volume (VEC) are
ultimately determined by the plasma volume. The interstitial fluid volume
(VIF) is calculated in a similar way.
The Na, K and Cl concentrations
The sodium concentration in the extracellular fluid (XNE) depends on
the quantity of water in the extracellular compartment (VEC) and the absolute
quantity of sodium (ZNE). The absolute quantity of sodium is, among others,
a function of the mean average daily sodium intake (YNIN) and the quantity
which leaves the body in the form of urine (YNU). The same holds true for
potassium (XKE, ZKE, YKIN, YKU) and chlorine (XCLE, ZCLE, YCLI, YCLU) .
The model is similarly built for calcium, magnesium, glucose, bicarbonate,
phosphate, sulphate, urea, mannitol, other organic acids, and proteins.
Kidney and urine
The osmolality of urine (OSMU) is determined by the urine output (QWU),
the glomerular filtration velocity (GFR) and the antidiuretic hormone (ADH).
The pH of the urine (PHU) is calculated a.o. from the renal clarification
velocity of organic salts (YORG) and phosphate (YPO4).
The glomerular filtration velocity
The glomerular filtration velocity (GFR) is only a function of the
mean arterial pressure (PAS) and the extra-cellular fluid volume (VEC)
here.
Respiration
The ventilation (VI) is determined by a function (F) of the CO2 pressure
(PCOA), the O2 pressure in the alveoli (PO2A) and the pH of the arterial
blood (PHA). The pH in the alveoli (PHA) is calculated with the Henderson-Hasselbalch
equation.
These values are only valid when no interventions have taken place in
the model and one can speak of a steady state. In the casuistry here described
in respect of thirsting, sweating, drinking, and infuses other variables
often play an important role. Therefore it is also possible to measure
and register other arbitrary variables. If some of these variables exceed
or fall below a critical value, the students will be warned by a message.
The symptoms appearing in the computer simulation program FLUIDS are
determined by a range of values. As soon as they come above or below a
certain level, a message is given to the student:
Thirsting, sweating and loss of salt
An important simulation is to withhold water from the testee (the model)
over an extended period. When there is also loss of fluid (QIWI) and much
loss of salt (via YNIN and YCLI) together with the thirsting, as can be
seen in Figure 10.3. During the simulation time the water intake is QIN
= 0 l/min and the velocity with which fluid is withdrawn (QWIL) from the
body has increased from 0.007 l/min to 0.008 l/min (which is equal to 0.5
l/hour). The sodium and chlorine intake (YNIN and YCLI), which are normally
0.12 respectively 0.133 mEq/min, are both fixed on -1.2 mEq/min. It can
clearly be seen that the ADH increases and the urine excretion reduces
sharply. The blood pressure (PAS) falls, in contrast with the situation
of drinking pure water only in which the osmolality of plasma and urine
increases slightly.
Water intake: drinking, water-infuse or physiological salt infuse
Water intake can be simulated by the model in three ways: drinking,
hypertonic infuse or a physiological salt infuse.
Figures 10.4 shows the consequences of intravenous injection of one
litre of physiological salt in five minutes, while the sodium (YNIN) and
intake are both 31 mEq/min. This is equal to a quantity of sodium and chlorine
of 154 mEq. The figure shows how the model reacts with a rise in blood
pressure (PAS). Here also the kidney will act as a regulator: due to the
rise in blood pressure the kidney will excrete salt. This lasts for several
hours. When the salt is being excreted the water excretion will follow.
Min, F.B.M., (1996)
Simulation Technology & Parallelism in Learning Environments; Methods, Concepts,
Models and Systems. Publisher: Academic Book Center, De Lier. ISBN 90-5478-036-3
Min, F.B.M., (1993)
Biomedical Modelling and simulation on a PC; A Workbench for Physiology and Biomedical Engineering (3 chapters). Springer-Verlag New York Berlin; (Editors: R. van Wijk van Brievingh and D. Müller).
Note
The first version of this chapter was first published in Dutch in:
Min 1982; Ph.D thesis, University of Limburg, Maastricht and in English
in: D. Möller and R. Van Wijk van Brievingh (editors), Springer Verlag,
Berlin.
Note
The complete model listing in Fortran is given by R. Van Wijk van Brievingh
and D. Möller (1993). In this book an executable MS.DOS version on
floppy of FLUIDS has been enclosed. There is also nowe a listing in Pascal. We are working also for a source writen in Java now.
Note about the figures:
See them all of better quality in the real existing book: Rik Min, Simulation Technology & Parallelism in Learning Environments; Methods, Concepts, Models and Systems. Publisher: Academic Book Center, De Lier. Holland. ISBN 90-5478-036-3 (1996).
Acknowledgement
The author gratefully acknowledges the contribution of Dr. Ikeda and
his co-workers at Kitasato University, Japan, to the computer simulation
program FLUIDS.
